Adjoint representation of a Lie group

Every Lie group have a canonical group representation.

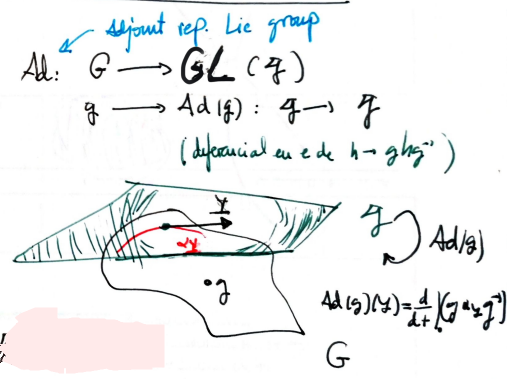
Given a Lie group $G$ , every $g \in G$ determines a smooth group homomorphism of $G$ onto itself, $C_{g} \in Aut (G)$ , by means of the assignation

L_{g} \circ R_{g^{- 1}} : h \mapsto g h g^{- 1}

If we consider the differential of this map at the identity we can define:

A d_{g} := d (L_{g} \circ R_{g^{- 1}})_{e} : T_{e} G ≅ g ⟶ T_{e} G ≅ g

And so we have defined an morphism $G ⟼ G L (g)$ , and we have a group representation of $G$ .

Explicitly, and taking into account the exponential map, we have

\begin{array}{rcl} A d_{g} : g & ⟶ & g \\ V & ⟶ & \frac{d}{d t} (g e^{t V} g^{- 1}) |_{t = 0} \end{array}

Idea

Given $V \in g$ , we can think of it like a curve $α (t)$ emanating from $e \in G$ with velocity $V$ . Then, for $g \in G$ we have another curve $β (t) = g α (t) g^{- 1}$ . The assignation

V \mapsto β^{'} (0)

is a linear map from $g$ to $g$ , that is, a representation.

To _see the action_ of $g$ over $V$ think of the action of $g$ over any space $M$ it acts (you can choose the proper $G$ ). In a point $P \in M$ you can act with $V$ to begin a little curve that arrive to a very near point $Q$ . But you can, instead, begin at $P$ , go back to $g^{- 1} P$ , start the little curve produced with $h$ and apply $g$ to the final point. You will have obtained a another point different from $Q$ , say $Q^{'}$ . The little curve starting at $P$ toward $Q^{'}$ is that produced by $g V g^{- 1}$ .

Ad(G)-module structure

Since $A d (G) = {A d_{g} : g \in G}$ is a subring of $E n d (g)$ then $g$ has the structure of a $A d (G)$ -module.

If $H$ is a subgroup of $G$ then it has also the structure of a $A d (H)$ -module.

Adjoint representation of a Lie algebra

Given the adjoint representation of the Lie group $G$ as above, we have

A d : G \to G L (g)

which is a Lie group homomorphism. So we can think of the differential at identity

d (A d)_{e} : T_{e} G \to T_{i d} G L (g),

expression that, after defining $a d := d (A d)_{e},$ can be rewritten as

a d : g \to gl (g)

Explicitly, given $X, Y \in g$ , and by using the exponential map

a d (X) (Y) = \frac{d}{d s} |_{s = 0} (A d_{e^{s X}} (Y)) = \frac{d}{d s} |_{s = 0} (A d_{e^{s X}} (\frac{d}{d t} |_{t = 0} e^{t Y})) =

= \frac{d}{d s} \frac{d}{d t} |_{s, t = 0} (e^{s X} e^{t Y} e^{- s X})

This expression can be simplified even further. Since $a d (X) (Y) \in T_{e} G$ consider a smooth function $f$ defined in an open neighbourhood of $e \in G$ . Then

\begin{matrix} (1) & a d (X) (Y) (f) = \frac{d}{d s} \frac{d}{d t} |_{s, t = 0} (f (e^{s X} e^{t Y} e^{- s X})) \end{matrix}

Now, observe that if $F (t_{1}, t_{2})$ is a function of two real variables, by the chain rule

\frac{d}{d s} |_{s = 0} F (s, - s) = \partial_{t_{1}} F (t_{1}, 0) |_{t_{1} = 0} - \partial_{t_{2}} F (0, t_{2}) |_{t_{2} = 0}

So now, if we fix $t$ in $(1)$ and apply the chain rule to $s$ we obtain

\frac{d}{d t} |_{t = 0} (\frac{d}{d s} |_{s = 0} f (e^{s X} e^{t Y}) - \frac{d}{d s} |_{s = 0} f (e^{t Y} e^{s X}))

Remember that for a smooth function $g$ and $\hat{X}$ a left invariant vector field extending $X \in g$

\frac{d}{d s} |_{s = 0} (g (h e^{s X})) = \hat{X} (g) (h)

(see exponential map). Therefore

\frac{d}{d t} |_{t = 0} \frac{d}{d s} |_{s = 0} f (e^{s X} e^{t Y}) = \frac{d}{d s} |_{s = 0} (\hat{Y} (f) (e^{s X})) =

= \hat{X} (\hat{Y} (f) (e))

and

\frac{d}{d t} |_{t = 0} \frac{d}{d s} |_{s = 0} f (e^{t Y} e^{s X}) = \frac{d}{d t} |_{t = 0} (\hat{X} (f) (e^{t Y})) =

= \hat{Y} (\hat{X} (f) (e))

and so

a d (X) (Y) (f) = [X, Y] (f) .

(Reference: Bump, Lie Groups, proposition 8.2, page 49.)

Finally, I think that the adjoint representation of a Lie algebra is a particular case of Lie algebra action.

________________________________________

Author of the notes: Antonio J. Pan-Collantes

antonio.pan@uca.es

INDEX: